- Chart Creator
- Ayanamshas in Sidereal Astrology
- Free Online Lahiri Ayanamsa To Calculate The Precession
However, the historical basis of this ayanamsha is problematic and many experts consider it wrong by several degrees. However the official definition of the Lahiri ayanamsha does not realise this idea accurately. This is explained by the fact that the traditional method of calculating ayanamshas does not take into account the proper motion of fixed stars and a small change in orientation of the ecliptic plane.
For this reason, some astrologers have proposed an improved version of this ayanamsha, the so-called "True Chitra Paksha Ayanamsha". This ayanamsha is considered to be a correction of the Lahiri ayanamsha. It must be noted, however, that this work does not talk about ayanamsha, but only mentions the positions of some fixed stars in a sidereal zodiac.
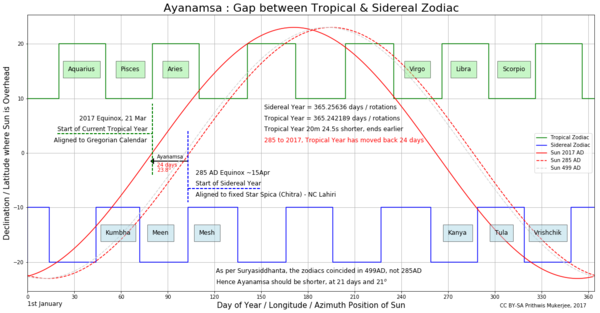
In its present form, the Suryasiddhanta was composed near the year CE. Older versions of it are not extant, but are referred to in other texts. In polar projection, the projection line is not perpendicular to the ecliptic but is drawn through the celestial north pole and the star.
Since the above-mentioned Spica-based ayanamshas Lahiri und True Chitra are projected on the ecliptic in a right angle, strictly speaking they cannot be justified on the basis of the Suryasiddhanta. For this reason, this ayanamsha should not be considered very reliable either. The ayanamsha used by the astrologer K.
Krishnamurti is close to the Lahiri ayanamsha and the True Chitra Ayanamsha.
Since ancient Indian astronomers were not aware of the galactic centre, this coincidence seems to be rather accidental.
From a philosophical point of view, it probably makes a lot more sense to fix the sidereal zodiac at the galactic centre than at some random fixed star. Since all visible stars circle around the galactic centre, it could be called the "central star" of our galaxy.
The galactic centre is also millions of times heavier than any star. Two different ayanamshas can be derived from this information. With the True Revati Ayanamsha , the star is assumed at this ecliptic position in rectangular projection.
With the Suryasiddhanta Revati Ayanamsha , on the other hand, the zodiac is defined in such a way that Revati had the same position in polar ecliptic projection in the year CE. The latter seems to be more appropriate because the Suryasiddhanta uses polar projection.
However, it must be noted that the position of Revati as given in the Suryasiddhanta is incompatible with the positions of Spica and other stars as given in the same work. Unfortunately, the star positions of the Suryasiddhanta do not allow us to determine the underlying ayanamsha.
This ayanamsha is named after two authors called Usha and Shashi. The galactic centre is a massive black hole in the centre of the Milky Way. Our Sun and all visible stars circle around it.
This ayanamsha was introduced in by the American astrologer Ernst Wilhelm. The galactic centre is projected on the ecliptic in polar projection, i. The point at which this great circle cuts the ecliptic is defined as the middle of the nakshatra Mula.
This ayanamsha is very close to the Usha-Shashi ayanamsha and the Revati ayanamshas. This ayanamsha is a little less stable than the other ayanamshas. Since polar projection is used, it is a little bit influenced by general precession.
Chart Creator
However, polar projection was actually the method used by the Suryasiddhanta. It must be noted that Wilhelm uses this ayanamsha only for the definition of the nakshatra circle not for the zodiac, because he uses the tropical zodiac combined with sidereal nakshatras.
More information on this ayanamsha is found in the General Documentation of the Swiss Ephemeris. This ayanamsha, which is close to the Raman ayanamsha, was proposed by the German-Spanish astrologer Rafael Gil Brand Rafael Gil Brand, Himmlische Matrix. This ayanamsha was used by the great Indian astrologer Bangalore Venkata Raman Although this ayanamsha is very close to the galactic ayanamsha of Gil Brand, Raman apparently did not think of the possibility to define the zodiac using the galactic centre.
Raman, Hindu Predictive Astrology , pp. Here, the year CE is given as the year of zero ayanamsha. This ayanamsha was allegedly recommended by Swami Shri Yukteshwar Giri We have taken over its definition from Graham Dawson.
However, the definition given by Yukteshwar himself in the introduction of his work The Holy Science is a confusing. At the same time he believed that this was the distance of the spring equinox from the star Revati, which he put at the initial point of Aries.
Moreover, Yukteshwar is wrong in assigning the above-mentioned ayanamsha value to the year ; in reality it applies to Since Yukteshwar's precession rate is wrong by 4" per year, astro.
In , the difference amounts to 6'40". Although this ayanamsha differs only a few arc seconds from the galactic ayanamsha of Gil Brand, Yukteshwar obviously did not intend to define the zodiac using the galactic centre. He actually intended a Revati-oriented ayanamsha, but committed the above-mentioned errors in his calculation.
This ayanamsha was proposed by the Indian astrologer P. Narasimha Rao, the author of the astrological software Jagannatha Hora. He argues that the human existence has its root in the heart, which corresponds to the sign of Cancer. Narasimha Rao, "Introducing Pushya-paksha Ayanamsa" This ayanamsha ist derived from ancient Indian time cycles and astronomical information given in the Mahabharata.
Sunil Sheoran, therefore calls this ayanamsha "Vedic". Essential in Sheoran's argumentation is the assumption that the two Mahabharatan solar eclipses that were observed from Kurukshetra and Dvaraka were 18 years apart, not 36 years as is taught by tradition and the Mahabharata itself.
From the mentioned eclipse pair and historical considerations, he derives that the Mahabharata war must have taken place in the year BCE.
Then he dates the beginning of the last Manvantara on the winter solstice BCE. Unfortunately, there are serious problems at least in Sheoran linguistic argumentation. As to the time distance between the two eclipses, the Mahabharata itself states: Unfortunately, Sanskrit dictionaries and grammar do not allow such translations.
This ayanamsha was calculated in by Peter Huber, a Swiss expert in Babylonian mathematics and astronomy, based on a statistical investigation of cuneiform astronomical tablets. Centaurus , 5, p. This ayanamsha was calculated in by the American astronomy historian John P. Britton based on a statistical investigation of cuneiform astronomical tablets.
It is an attempt to improve P. Britton, "Studies in Babylonian lunar theory: The introduction of the uniform zodiac", in Arch.
Ayanamshas in Sidereal Astrology
He refers to the doctrine of the Kalapurusha which assigns the 12 zodiac signs to parts of the human body. The initial point of Aries is considered to correspond to the crown and Pisces to the feet of the cosmic human being.
In addition, Chandra Hari notes that Mula has the advantage to be located near the galactic centre and to have "no proper motion". Chandra Hari believes it defines the original Babylonian zodiac.
As has been stated, the position of the galactic centre was not known to the ancient peoples. However, they were aware of the fact that the Milky Way crossed the ecliptic in this region of the sky.
Indian Journal of History of Science, 33 4 A few astrologers have started to fix a sidereal-like zodiac at the "galactic nodes", i. This kind of solution as well as ayanamshas oriented towards the galactic centre are obviously more convincing from a philosophical point of view, because the galaxy is the greater whole, within which our Sun and all visible stars move and exist.
Mardyks' view, this kind of ayanamsha or zodiac is not sidereal, but galactic. Nevertheless, it is as "fixed" as a sidereal zodiac. This ayanamsha was proposed in by the American astrologer Raymond Mardyks.
The autumnal equinoctial point was conjunct the north galactic pole and the solstices were conjunct the galactic nodes. A similar alignment occurs four times in a full precessional cycle. In Mardyks' view, this galactically aligned zodiac and ayanamsha opens astrology to higher "galactic" dimensions which are also considered in Maya astrology.
This ayanamsha or zodiac therefore has a "tropical" component. Mardyks calls it a "hybrid fixed-tropical, galactic zodiac". In astrological practice, Mardyks uses this galactic zodiac combined with the tropical zodiac along with the stars that compose the constellations. Mardyks' calculation is partially based on the galactic coordinate system that was defined by the International Astronomical Union in The Mountain Astrologer Aug.
This ayanamsha differs from the Skydram ayanamsha by only 19 arc seconds. The last two ayanamshas are based on a slightly outdated position of the galactic pole that was determined in According to more recent observations and calculations from the year , the galactic node with the ecliptic shifts by 3'11", and the "Galactic Alignment" is preponed to Mardyks still gives preference to the older galactic pole and plane, which are still used in astronomy as the standard galactic coordinate system.
This ayanamsha was introduced by the American astrologer Ernst Wilhelm in He used a calculation of the galactic node by D.
Koch from the year , which had a small error of 2 arc seconds. The current implementation of this ayanamsha is based on a new position of the Galactic pole found by Chinese astronomers in This ayanamsha was proposed by the American astrologer Robert DeLuce It is fixed at the birth of Jesus, theoretically at 1 January 1 AD.
DeLuce believes that this ayanamsha was also used in ancient India. He draws this conclusion from the fact that the important ancient Indian astrologer Varahamihira, who assumed the solstices on the ingresses of the Sun into sidereal Cancer and Capricorn, allegedly lived in the 1st century BC.
This dating of Varahamihira has recently become popular under the influence of Hindu nationalist ideology Hindutva. However, historically, it cannot be maintained. Varahamihira lived and wrote in the 6th century AD. This ayanamsha is based on the assumption that the Age of Aquarius will begin in the year This assumption is maintained by a theosophical society called Ageless Wisdom , and bases itself on a channelled message given in by a certain spiritual master called Djwhal Khul.
The ancient Indian astronomer Aryabhata states that from the beginning of the Kaliyuga Kali Age in BCE until the spring equinox CE Aryabhata's own 23rd year of life exactly years have passed.
In addition, he assumes the spring equinox at the initial point of Aries. From this information, two possible ayanamshas can be derived.
Either the zero point of the zodiac is assumed at the position of the equinoctial point on the spring equinox CE, or otherwise at the position of the mean Sun exactly sidereal years after the beginning of the Kaliyuga. More information on these ayanamshas is found in the General Documentation of the Swiss Ephemeris.
These ayanamshas are calculated using the same methods as the two Aryabhata ayanamshas above, however using the year length of the Suryasiddhanta. According to Govindasvamin n. This tradition probably goes back to an erroneous interpretation of Aryabhata's above-mentioned statement that he was 23 years old when had elapsed after the beginning of the Kaliyuga.
These ayanamshas are not used in astrology. In fact, they are not ayanamshas at all, but astronomical sidereal coordinate systems, where the tropical ecliptic of the beginning of the year , , or is defined as a sidereal reference frame. Ayanamshas are usually defined using the positions of certain fixed stars.
The following fixed stars played an important role in the history of the zodiac:. Unfortunately, nobody can tell why any of these stars should be so important that it could be used as an anchor point for the zodiac. In addition, all these solutions are unattractive in that the fixed stars actually are not fixed forever, but have a small proper motion which over a long period of time such as several millennia, can result in a considerable change in position.
The appearance of the sky changes over long periods of time. In ' years, the constellation will look very different from now, and the nakshatras lunar mansions will get confused. For this reason, a zodiac defined by positions of stars is unfortunately not able to provide an everlasting reference frame.
Constellations of the zodiac around the year ' BC. If one were to travel back to that time, one could not easily recognise any of the constellations except Orion. Incidentally, this phenomenon not only challenges current definitions of ayanamsha, which anchor the zodiac at some fixed star, but also obviously proves that the zodiacal constellations either have no reality and are mere imagination or otherwise that they are a transient and perishable thing.
In addition, it is obvious that the astrological zodiac of 12 equal signs with all its wonderful internal logic and symmetry, if it is real at all and an everlasting archetype of the cycles of life, cannot derive its effectiveness from a random distribution of unrelated fixed stars, but must be based on something more stable and more fundamental.
For such or also other reasons, some astrologers Raymond Mardyks, Ernst Wilhelm, Rafael Gil Brand, Nick Anthony Fiorenza have tried to redefine the sidereal zodiac using either the galactic centre or the node of the galactic equator with the ecliptic. It is obvious that this kind of solution, which would not depend on the position of a single star anymore, could provide a philosophically meaningful and very stable definition of the zodiac.
Fixed stars would be allowed to change their positions over very long periods of time, but the zodiac could still be considered fixed and "sidereal". Disregarding historical considerations for a moment, it would be philosophically convincing to define the sidereal zodiac relative to the galactic centre, around which our Sun and all visible stars circle.
Assuming the galactic centre here seems to make sense. Kar on Chitra paksha Ayanamsa. Sri Lahiri and Professor Vaidya pointed out that if any change is introduced in the ayanamsa at this stage, The calendar for Four years so far calculated will require a thorough revision involving a great amount of labour and time.
It was, however, agreed that if the difference be small such as one or two minutes of arc, the labour involved in the revision would not be much.
C Lahiri Ayanamsa is correct, then why did Sri N. C Lahiri agree to change one or two minutes of Arc in his Ayanamsa? Why did he mention about Labour and recalculation of Panchangas?
Since the vernal equinox point precesses westwards at a rate of about 50". On the other hand, since the stars "do not move" this ignores the effect of proper motion the longitude of a fixed body defined with respect to them will never change. Traditional Vedic astrology Jyotisha uses a system of sidereal longitude.
When the practitioners of these schools of astrology use modern astronomical calculations to determine the position of celestial bodies, they need to take into account the difference caused by the different reference point used in specifying the longitude, and this they call the ayanamsa.
Some orthodox schools of Vedic astrology reject modern astronomy and still base their computations upon traditional texts and treatises, mostly following the Surya Siddhanta or treatises based on it. In West Theon ca. The ayanamsha describes the increasing gap between the tropical and sidereal zodiacs. Western Astrologers Fagan and Bradley computed it at 24 degrees in ; however, there are various values in use in India.
While the general consensus among Western siderealists is that the star Alcyon represents the first point of Aries, differences arise because of the indefinite ancient boundaries of the constellation of Aries.
Indian definition of astrological signs is not based on constellations but on equal angular division of sky, which makes it difficult to define signs in terms of stars and constellations. This is the source of controversy about ayanamsha. From Wikipedia, the free encyclopedia. This article has multiple issues.
Free Online Lahiri Ayanamsa To Calculate The Precession
Please help improve it or discuss these issues on the talk page. Learn how and when to remove these template messages. This article needs attention from an expert on the subject.
Please add a reason or a talk parameter to this template to explain the issue with the article. When placing this tag, consider associating this request with a WikiProject.